If I_1, I_2, I_3 and I_4 are the respective r | vedclass

Name: Exam Paper Generator | JEE NEET Paper Generator | Vedclass
Brand: Vedclass
Rating: 5 (35 reviews)

Question

(B) The $r.m.s.$ value of a current is defined as $I_{rms} = \sqrt{\frac{1}{T} \int_0^T i^2 dt}$.For case $I$ and $II$,the current is a rectified sine

Vedclass · Accepted Answer

$I_3 > I_1 = I_2 > I_4$

Vedclass · Answer

(B) The $r.m.s.$ value of a current is defined as $I_{rms} = \sqrt{\frac{1}{T} \int_0^T i^2 dt}$.For case $I$ and $II$,the current is a rectified sine wave: $i = |I_0 \sin(\omega t)|$. The $r.m.s.$ value is $I_1 = I_2 = \frac{I_0}{\sqrt{2}} \approx 0.707 I_0$.For case $III$,the current is a square wave: $i = I_0$ for half cycle and $-I_0$ for the other half. The $r.m.s.$ value is $I_3 = \sqrt{\frac{1}{T} (I_0^2 \cdot \frac{T}{2} + (-I_0)^2 \cdot \frac{T}{2})} = I_0$.For case $IV$,the current is a triangular wave: $i = \frac{2I_0}{T} t$ for $0 Comparing the values: $I_3 = I_0$,$I_1 = I_2 = 0.707 I_0$,and $I_4 = 0.577 I_0$.Thus,the correct relation is $I_3 > I_1 = I_2 > I_4$.

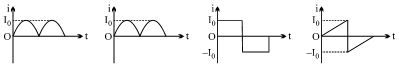
If $I_1, I_2, I_3$ and $I_4$ are the respective $r.m.s.$ values of the time-varying currents as shown in the four cases $I, II, III$ and $IV$,then identify the correct relation.

Similar Questions

What is the average value of the $AC$ voltage over a complete cycle?

An $AC$ source rated $220\, V, 50\, Hz$ is connected to a resistor. The time taken by the current to change from its maximum value to the $rms$ value is:

$A$ current in a circuit is given by $i = 3 + 4 \sin \omega t$. The effective value of the current is

In India,$AC$ voltage becomes zero how many times in $1$ $sec$?

The instantaneous values of current and voltage in an $AC$ circuit are given by $I = 6 \sin(100 \pi t + \frac{\pi}{4})$ and $V = 5 \sin(100 \pi t - \frac{\pi}{4})$. Then,

Vedclass Test Series

Exam Paper Generator

Online Exam Module