Consider a parallel plate capacitor of $10\,\mu \,F$ (micro-farad) with air filled in the gap between the plates. Now one half of the space between the plates is filled with a dielectric of dielectric constant $4$, as shown in the figure. The capacity of the capacitor changes to.......$\mu \,F$
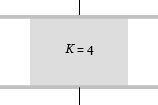
Consider a parallel plate capacitor of $10\,\mu \,F$ (micro-farad) with air filled in the gap between the plates. Now one half of the space between the plates is filled with a dielectric of dielectric constant $4$, as shown in the figure. The capacity of the capacitor changes to.......$\mu \,F$

- A
$25$
- B
$20$
- C
$40$
- D
$5$
Similar Questions
A parallel plate capacitor is made of two square plates of side $a$, separated by a distance $d\,(d < < a)$. The lower triangular portion is filled with a dielectric of dielectric constant $K$, as shown in the figure. Capacitance of this capacitor is
A parallel plate capacitor is made of two square plates of side $a$, separated by a distance $d\,(d < < a)$. The lower triangular portion is filled with a dielectric of dielectric constant $K$, as shown in the figure. Capacitance of this capacitor is
- [JEE MAIN 2019]
A parallel plate air capacitor has a capacitance of $100\,\mu F$. The plates are at a distance $d$ apart. If a slab of thickness $t(t \le d)$and dielectric constant $5$ is introduced between the parallel plates, then the capacitance will be.......$\mu F$
A parallel plate air capacitor has a capacitance of $100\,\mu F$. The plates are at a distance $d$ apart. If a slab of thickness $t(t \le d)$and dielectric constant $5$ is introduced between the parallel plates, then the capacitance will be.......$\mu F$
The capacitance of a parallel plate capacitor is $C$ when the region between the plate has air. This region is now filled with a dielectric slab of dielectric constant $k$. The capacitor is connected to a cell of $emf$ $E$, and the slab is taken out
The capacitance of a parallel plate capacitor is $C$ when the region between the plate has air. This region is now filled with a dielectric slab of dielectric constant $k$. The capacitor is connected to a cell of $emf$ $E$, and the slab is taken out
A parallel - plate capacitor with plate area $A$ has separation $d$ between the plates. Two dielectric slabs of dielectric constant ${K}_{1}$ and ${K}_{2}$ of same area $\frac A2$ and thickness $\frac d2$ are inserted in the space between the plates. The capacitance of the capacitor will be given by :
A parallel - plate capacitor with plate area $A$ has separation $d$ between the plates. Two dielectric slabs of dielectric constant ${K}_{1}$ and ${K}_{2}$ of same area $\frac A2$ and thickness $\frac d2$ are inserted in the space between the plates. The capacitance of the capacitor will be given by :
- [JEE MAIN 2021]
A capacitor of capacity $'C'$ is connected to a cell of $'V'\, volt$. Now a dielectric slab of dielectric constant ${ \in _r}$ is inserted in it keeping cell connected then
A capacitor of capacity $'C'$ is connected to a cell of $'V'\, volt$. Now a dielectric slab of dielectric constant ${ \in _r}$ is inserted in it keeping cell connected then
