The graph of position $x$ versus time $t$ represents the motion of a particle. If $b$ and $c$ are both positive constants, which of the following expressions best describes the acceleration $a$ of the particle?
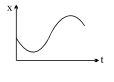
The graph of position $x$ versus time $t$ represents the motion of a particle. If $b$ and $c$ are both positive constants, which of the following expressions best describes the acceleration $a$ of the particle?

- A
$a = b - ct$
- B
$a = + b$
- C
$a = - c$
- D
$a = b + ct$
Similar Questions
The coordinates of a moving particle at any time are given by $x = a{t^2}$ and $y = b{t^2}$. The speed of the particle at any moment is
The coordinates of a moving particle at any time are given by $x = a{t^2}$ and $y = b{t^2}$. The speed of the particle at any moment is
A vector has magnitude and direction. Does it have a location in space ? Can it vary with time ? Will two equal vectors $a$ and $b$ at different locations in space necessarily have identical physical effects ? Give examples in support of your answer.
A vector has magnitude and direction. Does it have a location in space ? Can it vary with time ? Will two equal vectors $a$ and $b$ at different locations in space necessarily have identical physical effects ? Give examples in support of your answer.
An aircraft is flying at a height of $3400\; m$ above the ground. If the angle subtended at a ground observation point by the aircraft positions $10.0\; s$ apart is $30^o$, what is the speed in $m/s$ of the aircraft ?
An aircraft is flying at a height of $3400\; m$ above the ground. If the angle subtended at a ground observation point by the aircraft positions $10.0\; s$ apart is $30^o$, what is the speed in $m/s$ of the aircraft ?
A body starts from rest from the origin with an acceleration of $6\,m/{s^2}$ along the $x-$axis and $8\,m/{s^2}$ along the $y-$axis. Its distance from the origin after $4 \,seconds$ will be........$m$
The displacement of a particle from a point having position vector $2 \hat{i}+4 \hat{j}$ to another point having position vector $5 \hat{i}+1 \hat{j}$ is ........ units
The displacement of a particle from a point having position vector $2 \hat{i}+4 \hat{j}$ to another point having position vector $5 \hat{i}+1 \hat{j}$ is ........ units
