$STATEMENT-1$ A vertical iron rod has a coil of wire wound over it at the bottom end. An alternating current flows in the coil. The rod goes through a conducting ring as shown in the figure. The ring can float at a certain height above the coil. Because
$STATEMENT- 2$ In the above situation, a current is induced in the ring which interacts with the horizontal component of the magnetic field to produce an average force in the upward direction.
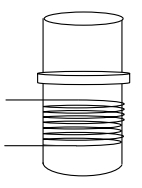
$STATEMENT-1$ A vertical iron rod has a coil of wire wound over it at the bottom end. An alternating current flows in the coil. The rod goes through a conducting ring as shown in the figure. The ring can float at a certain height above the coil. Because
$STATEMENT- 2$ In the above situation, a current is induced in the ring which interacts with the horizontal component of the magnetic field to produce an average force in the upward direction.

- [IIT 2007]
- A
Statement -$1$ is True, Statement-$2$ is True; Statement -$2$ is a correct explanation for Statement-$1$.
- B
Statement -$1$ is True, Statement-$2$ is True; Statement -$2$ is $NOT$ a correct explanation for Statement-$1$.
- C
Statement -$1$ is True, Statement- $2$ is False.
- D
Statement -$1$ is False, Statement-$2$ is True.
Similar Questions
The horizontal component of earth's magnetic field at a place is $3.5 \times 10^{-5} \mathrm{~T}$. A very long straight conductor carrying current of $\sqrt{2} A$ in the direction from South east to North West is placed. The force per unit length experienced by the conductor is$..............$ $\times 10^{-6} \mathrm{~N} / \mathrm{m}$.
The horizontal component of earth's magnetic field at a place is $3.5 \times 10^{-5} \mathrm{~T}$. A very long straight conductor carrying current of $\sqrt{2} A$ in the direction from South east to North West is placed. The force per unit length experienced by the conductor is$..............$ $\times 10^{-6} \mathrm{~N} / \mathrm{m}$.
- [JEE MAIN 2024]
Assertion : Free electrons always keep on moving in a conductor even then no magnetic force act on them in magnetic field unless a current is passed through it.
Reason : The average velocity of free electron is zero.
Assertion : Free electrons always keep on moving in a conductor even then no magnetic force act on them in magnetic field unless a current is passed through it.
Reason : The average velocity of free electron is zero.
- [AIIMS 2016]
Two parallel wires in free space are $10\, cm$ apart and each carries a current of $10\, A$ in the same direction. The force one wire exerts on the other per metre of length is
Two parallel wires in free space are $10\, cm$ apart and each carries a current of $10\, A$ in the same direction. The force one wire exerts on the other per metre of length is
- [AIPMT 1997]
Three long, straight and parallel wires carrying currents are arranged as shown in the figure. The wire $C$ which carries a current of $5.0\, amp$ is so placed that it experiences no force. The distance of wire $C$ from wire $D$ is then
Three long, straight and parallel wires carrying currents are arranged as shown in the figure. The wire $C$ which carries a current of $5.0\, amp$ is so placed that it experiences no force. The distance of wire $C$ from wire $D$ is then
A square of side $2.0\,m$ is placed in a uniform magnetic field $B = 2.0\, T$ in a direction perpendicular to the plane of the square inwards. Equal current, $i = 3.0\, A$ is flowing in the direction shown in figure. Find the magnitude of magnetic force on the loop
A square of side $2.0\,m$ is placed in a uniform magnetic field $B = 2.0\, T$ in a direction perpendicular to the plane of the square inwards. Equal current, $i = 3.0\, A$ is flowing in the direction shown in figure. Find the magnitude of magnetic force on the loop
